Conic Sections

Tasks
Find a circle. Is this the only place to find a circle?
What other shapes can you discover?
When do the ellipses stop? Is there anything special about that angle?
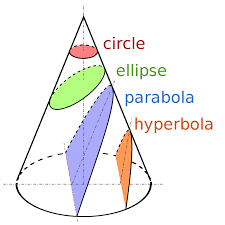
Maths

As you change the angle you will see the cross-section change. These are called conic sections. There are four types of conic section:

When the cone is vertical you will see a circle.

As the angle becomes steeper, the circle will change into an ellipse.

When the liquid is parallel to the side of the cone, we have a parabola. This this the curve a ball makes in the air.

Continue to make the angle steeper, and we will make hyperbola. This is the curve traced by a shadow on the ground throughout a day.
History

Conic sections were studied by the Ancient Greek mathematicians, including Euclid and Archimedes. Another Ancient Greek mathematician, called Apollonius of Perga, wrote eight books describing the different kinds of conic sections and their properties.
In the 11th Century, Persian mathematician Omar Khayyam, used the intersection of circles, ellipses, parabolas and hyperbolas to solve equations. And was the first to have a general method to solve cubic equations.
People
Apollonius of Perga 200BC
Apollonius was a Greek mathematician and astronomer. The mathematicians of ancient Greece studied the cross-sections of a cone (known as conic sections), which culminated in an extensive book by Apollonius on conic sections and their properties.

Omar Khayyam 1048 – 1131
Omar Khayyam was a mathematician from Persia (modern day Iran). Khayyam was famous for his work in geometry, astronomy and poetry. Khayyam reformed the Persian calendar which continued to be used for centuries. Khayyam invented a way to solve cubic equations using the intersection of conic sections.
Applications

The parabola is the curve a ball makes when thrown through the air. The shape is also used in antenna to pick up radio signals, or used in headlights to make a strong beam of light.

The hyperbola is the curve traced by a shadow on the ground during a day, and is used in the design of sundials.

In astronomy, gravity will cause a planet to orbit around a sun in an ellipse. On the other hand, if two objects are not in orbit, gravity will cause them to move in a parabola or hyperbola.
Maths at Home

Explore the cross sections of different shapes with these online demonstrations from GeoGebra and Interactive

However, if you want to get more hands-on, why not make the shapes yourself using play-doh. You can then (safely) slice the shapes and discover the cross sections inside.

See if you can you find all the cross sections in this table.